(1)
Indian Institute of Science Education and Research Pune (IISER-P), Pune, India
Abstract
After having traveled from ecology to physiology, endocrinology, metabolism, and immunology, we will return once again to the basic drawing board of ecology. Ecology and evolution are the basic foundations of all these arguments, and one should not lose touch with the foundation at any time. The dynamics of populations is a very fundamental process of ecology and evolution, and it is certainly very important in physiology and medicine too, but its importance is not appreciated sufficiently in these areas of science. Population level processes and individual’s behavior and physiology have subtle but far-reaching influences on each other, but this is an area that has not yet received sufficient attention. Ecologists have realized the importance of it [1–3] to some extent, but physiologists have not paid sufficient attention to it. I would attempt to make a case below for why and how population level processes are bound to affect individual behavior and physiology and why physiology and medicine need to understand population level processes. If I am able to convince the reader about this point, it would logically follow that today’s unprecedented density of human population is a supernormal stimulus that could change human behavior as well as physiology substantially, ultimately having some consequences for health.
After having traveled from ecology to physiology, endocrinology, metabolism, and immunology, we will return once again to the basic drawing board of ecology. Ecology and evolution are the basic foundations of all these arguments, and one should not lose touch with the foundation at any time. The dynamics of populations is a very fundamental process of ecology and evolution, and it is certainly very important in physiology and medicine too, but its importance is not appreciated sufficiently in these areas of science. Population level processes and individual’s behavior and physiology have subtle but far-reaching influences on each other, but this is an area that has not yet received sufficient attention. Ecologists have realized the importance of it [1–3] to some extent, but physiologists have not paid sufficient attention to it. I would attempt to make a case below for why and how population level processes are bound to affect individual behavior and physiology and why physiology and medicine need to understand population level processes. If I am able to convince the reader about this point, it would logically follow that today’s unprecedented density of human population is a supernormal stimulus that could change human behavior as well as physiology substantially, ultimately having some consequences for health.
One of the basic models of population growth is called the logistic model of growth which describes the dynamics of growth when the resources are finite. Initially developed to describe population growth in a simplistic way, different versions and refinements of the model have been used in different contexts often going beyond population growth and predicting other trends such as rates of discoveries in a scientific field or the learning curves [4–8]. The baseline model can be described by a single equation:
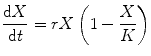 where X is the population size, r the intrinsic growth rate, and K the carrying capacity of the environment.
where X is the population size, r the intrinsic growth rate, and K the carrying capacity of the environment.
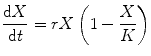
The equation is not difficult to interpret. The model has only two parameters, r, which is the intrinsic growth rate of the population, and K, which is the carrying capacity of the environment for the population of a given species. The term in the bracket denotes what fraction of the carrying capacity is yet unoccupied, and this gives a feedback to regulate the net growth rate. When the population is small, the feedback term is close to unity, and the equation simplifies to exponential growth. So when the population is small as compared to the carrying capacity of the environment, growth is nearly exponential. However, as the population increases, the feedback term starts becoming smaller and smaller which reduces the growth rate proportionately. When the population becomes equal to the carrying capacity, growth stops since the feedback term becomes zero. This dynamics results in a sigmoid growth curve (Fig. 9.1). The logistic curve forms the simple baseline model of population dynamics although in real life one sees situation-specific deviations from the assumptions of the model and the model can be refined to accommodate these complexities. For us, the baseline model is sufficient to build a conceptual framework.
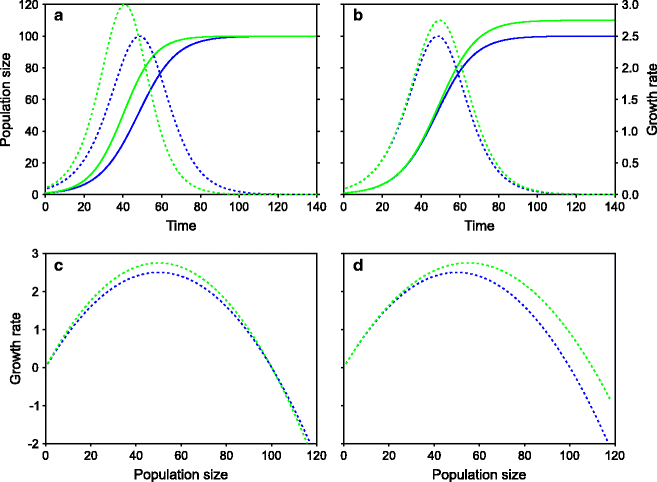

Fig. 9.1
The logistic growth curve: a fundamental model of population dynamics denoted by the equation 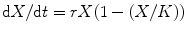 , where r indicates intrinsic growth rate of the population and K the carrying capacity of the environment. Solid lines indicate population sizes (primary Y axis), and dotted lines represent net growth rates (secondary Y axis). (a) The effect of r on population growth, the green line has 10% higher r. (b) The effect of K on population growth, the green line having 10% higher K. (c) and (d) The relationship between population density and growth rates: a population with a higher r (c) has higher growth rate at moderate population densities but loses this advantage at high densities. A population with higher K (d) has a higher net growth rate at population densities close to saturation. This is an important point to realize. A common misunderstanding about logistic growth model is that a higher r represents all-time advantage in growth rate. Near the carrying capacity, even a small increase in K can give a significant growth rate advantage
, where r indicates intrinsic growth rate of the population and K the carrying capacity of the environment. Solid lines indicate population sizes (primary Y axis), and dotted lines represent net growth rates (secondary Y axis). (a) The effect of r on population growth, the green line has 10% higher r. (b) The effect of K on population growth, the green line having 10% higher K. (c) and (d) The relationship between population density and growth rates: a population with a higher r (c) has higher growth rate at moderate population densities but loses this advantage at high densities. A population with higher K (d) has a higher net growth rate at population densities close to saturation. This is an important point to realize. A common misunderstanding about logistic growth model is that a higher r represents all-time advantage in growth rate. Near the carrying capacity, even a small increase in K can give a significant growth rate advantage
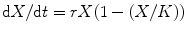 , where r indicates intrinsic growth rate of the population and K the carrying capacity of the environment. Solid lines indicate population sizes (primary Y axis), and dotted lines represent net growth rates (secondary Y axis). (a) The effect of r on population growth, the green line has 10% higher r. (b) The effect of K on population growth, the green line having 10% higher K. (c) and (d) The relationship between population density and growth rates: a population with a higher r (c) has higher growth rate at moderate population densities but loses this advantage at high densities. A population with higher K (d) has a higher net growth rate at population densities close to saturation. This is an important point to realize. A common misunderstanding about logistic growth model is that a higher r represents all-time advantage in growth rate. Near the carrying capacity, even a small increase in K can give a significant growth rate advantage
, where r indicates intrinsic growth rate of the population and K the carrying capacity of the environment. Solid lines indicate population sizes (primary Y axis), and dotted lines represent net growth rates (secondary Y axis). (a) The effect of r on population growth, the green line has 10% higher r. (b) The effect of K on population growth, the green line having 10% higher K. (c) and (d) The relationship between population density and growth rates: a population with a higher r (c) has higher growth rate at moderate population densities but loses this advantage at high densities. A population with higher K (d) has a higher net growth rate at population densities close to saturation. This is an important point to realize. A common misunderstanding about logistic growth model is that a higher r represents all-time advantage in growth rate. Near the carrying capacity, even a small increase in K can give a significant growth rate advantagePopulation ecologists and evolutionary biologists have used the model in different ways to cater for different classes of questions. While population ecologists have used it to predict growth trajectories, probabilities of extinctions, and effects of species interactions such as competition or predator prey and so on, evolutionary biologists have wondered how natural selection would shape the two parameters of the equation, namely, r and K. While it looks obvious that the population of a species with a higher reproductive rate r would grow faster and therefore outcompete one with smaller r, this is not unconditionally true. This can happen only under certain conditions. When much of the carrying capacity is still available so that the growth kinetics is close to exponential, a higher growth rate can give large returns. Therefore natural selection would favor larger r. However as the population approaches the carrying capacity, the importance of r is gradually reduced. Close to the carrying capacity, those that grow and reproduce faster are not the ones that have a larger r but those that make maximum efficient use of the limited resources available and ones that are highly competitive. In other words, those that make more efficient use of the limited resources have a higher effective K, and this is what selection will favor under crowded conditions. So at low population densities, there is selection for higher r, and at high population densities, there is selection for higher K. Then why can’t a genotype evolve that has a high r as well as high K so that it is the fittest genotype under all conditions? This does not happen because there is an inevitable trade-off between r and K. It is difficult to achieve both higher r and higher K simultaneously because the requirements for the two are conflicting and an individual has a finite resource. This has given rise to the concept of r and K selection [9–12].
In order to achieve a high reproductive rate, one has to increase the reproductive output. This can be done by increasing the number of offspring. Females can do this by increasing the number of eggs. Males can achieve this by mating with a greater number of females. However there is a cost in laying an extra egg or gaining an additional mating opportunity. Since every individual has a finite store of resources to be put in reproductive efforts, increasing the number of eggs might make reducing the size of eggs inevitable. A smaller egg may reduce the size and thereby the competitiveness of the emerging offspring. Competitiveness is an important component of K selection. Conversely, if egg size is kept large in spite of limited resources, either the number of eggs or longevity of the egg layer will have to be compromised. Perhaps both egg size and egg number may be increased by increasing the total fraction of resources going into reproduction. However, increasing reproductive effort might be accompanied by a compromise somewhere else such as longevity. This is an example of how there can be a conflict between r strategies of reproduction and K strategies of reproduction. It is difficult therefore to achieve both simultaneously. In reality, the precise form of expression of the r and K strategies and the nature of tradeoff is highly context dependent. For example, if saturating carrying capacity leads mainly to starvation, then a smaller body size and metabolic thrift could be the adaptive phenotype. However if it means increased aggressive competition, then a larger body size would be more adaptive. As a result, the characteristics that mark r and K strategies might be different in different species, but the robust principle is that the best reproductive strategies when a large fraction of carrying capacity is still available and those when it is saturated are different.
As a broad generalization, r strategy of reproduction is characterized by larger number of offspring with little investment in each and K by fewer offspring with greater investment in each. Some species are committed to the r strategy of reproduction and some others to the K strategy. Two extreme examples of r and K selection respectively are bacteria that may double the population every half an hour and elephants on the other hand that have a gestation period of 22 months ending in a single calf. Although the description so far sounds as if r and K are two discrete watertight compartments, in reality, they are the two ends of a continuum. Depending on the context, there can be an optimum position along the continuum that natural selection would favor. Originally the concept of r and K selection was applied across species, but now we know that even within a species, there can be a limited amount of variation between individuals with respect to their reproductive strategies [13–15]. Also for a given genotype, there could be some limited flexibility to shift towards r or towards K in one’s lifetime [16]. In crowded conditions, animals are expected to shift more towards K strategy. This flexibility is evident since fecundity is shown to be suppressed by crowding [17–20]. Under what conditions a species may evolve to have this plasticity in reproductive strategies?
We have seen that there is a feedback term in the logistic model which regulates the growth rate based on the available fraction of the carrying capacity. It is assumed in the baseline logistic model that this feedback is instantaneous. In reality, this does not work quite often. For example, as the population grows, there may be relative scarcity of food. This should result in reduced reproductive output. But there can be a substantial delay in this process. Often, the nutrition in early developmental period decides the adult reproductive potential causing a time delay in the cause and effect. There can be many other reasons for such a delay. If there is a delay in the feedback, the growth rate does not reduce sufficiently in time to stabilize the population at the carrying capacity. In such a case, the population may actually overshoot the carrying capacity. This makes the effective growth rate negative in the following generation(s). The population then declines until it is substantially below the carrying capacity and then starts rising again (Fig. 9.2). Such oscillations in populations are not uncommon in real life for a wide variety of species [21]. If the population of a species oscillates with considerable amplitude, the selective pressure for reproductive strategies would also be fluctuating. If we assume that r and K strategies are genetically determined, then when the population is at its peak, K reproductive strategies should be selected for, and when at its trough, r should be selected. This might result in a coexistence of r and K genotypes. The endocrinological and physiological requirements of the two reproductive strategies are likely to be substantially different, and therefore, one expects to see a different set of genes or gene expression patterns under the two states. But what if some genome has both the reproductive capacities and can shift its strategies appropriately as the population density changes? It is not very difficult to visualize that such plasticity will have a selective advantage over a wide range of amplitudes and frequencies of oscillations. For a species to have this plasticity, it should have the ability to shift between two endocrinological and metabolic states supporting the two strategies, and highly coordinated mechanisms should have evolved to attain such plasticity. Such plasticity would also help adapting to a fluctuating environment. Even if the population does not oscillate, environments are known to fluctuate widely, and different reproductive strategies may be best under different environmental states.
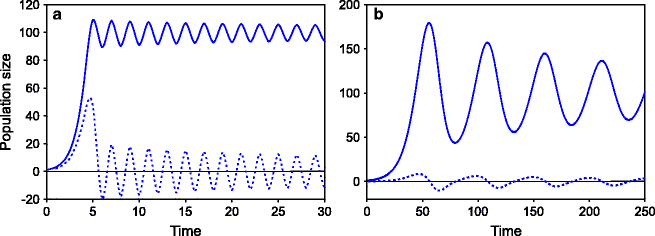

Fig. 9.2
Oscillations of populations around the carrying capacity: Populations can oscillate due to two main reasons. (a) If r is large, the oscillations are rapid typically completing one cycle in a few generations; (b) if there is a delay in feedback, there can be slower oscillations spanning over several generations. A combination of delayed sexual maturity and intrauterine programming of adult metabolic and reproductive functions can cause such a delay in feedback. Since both these factors are known to be predominant in humans, the prehistoric human populations are likely to have experienced (b)-type oscillations
We expect therefore that for a species which may have undergone population oscillations or wide environmental fluctuations for a sufficiently long evolutionary time, there could be plasticity in the reproductive strategy accompanied by a large number of coordinated changes in physiology. If such a phenomenon exists in the human species, it should be extremely relevant today since the human population is unprecedentedly high and therefore could serve as a supernormal stimulus for a K reproductive strategy.
We will now recall the insulin resistance syndrome and its associated sexual and reproductive states. The well-known consequences of insulin resistance for sex and reproduction are that on the one hand insulin resistance is associated with decreased ovulation [22–26] and decreased spermatogenesis [27–29] as well as subnormal sexual desire and function [30–35], all of which can result in reduced number of offspring. On the other hand, it is also well known that diabetic mothers have heavier babies [36–38]. This can be taken to mean that there is increased investment in the offspring whenever there is successful conception. The two effects together, namely, reduced chances of conception on the one hand and increased investment in offspring whenever conceived on the other, characterize K reproduction. We can suspect therefore that the reproductive component of insulin resistance syndrome is actually a K strategy of reproduction. The important question then is are the sexual and reproductive changes secondary to insulin resistance or are they the primary evolved plastic responses and is insulin resistance the accompanying physiological adjustment.
There appears to be evidence for both the directions. Type 1 diabetes in humans or streptozotocin-induced diabetes in rats results in sexual disorders indicating that diabetes comes first [30–35]. This is a more popular perception, and sexual dysfunction is perceived only as one of the many pathological consequences of diabetes. But there is also evidence on the contrary. Castration increased obesity and insulin resistance in rat as well as human studies [39, 40]. Contraceptives increase insulin resistance [41, 42]. Vasectomy induced atherogenic changes in some but not all primate studies [43–45] and fortunately not in humans [46, 47]. Erectile dysfunction is a common abnormality associated with diabetes [48–50]. Normalization of sugar does not appear to improve erectile dysfunction [51], but long-term treatment of erectile dysfunction with sildenafil is insulin sensitizing and restoring vascular function [52–55]. Diabetes does not affect menopause [56], but menopause leads to altered body fat distribution, impaired glucose tolerance, endothelial dysfunction, and systemic inflammation, all markers of insulin-resistant state [57]. These findings make us suspect that suppression of sex and reproduction could have a causal and not only a consequential role. For an evolutionary biologist, this certainly makes sense and is another example of an autocatalytic or positive feedback loop indicating a behavioral switch. If the possible number of offspring is reduced by any reason, the investment in each of the residual number of offspring should go up, and insulin resistance is the mechanism of doing so. On the other hand, if insulin resistance is primary and inevitably results in increasing the transplacental investment, it should be accompanied by a reduction in offspring number. The ultimate reasoning suggests that the association should be robust irrespective of the direction of the arrow of causation.
The other side of the story, that of diabetic mothers having heavier babies, is long known and undisputed. The mechanism by which this happens is also quite straightforward. Placental glucose uptake is insulin independent. Therefore, when maternal tissues develop insulin resistance, more glucose is diverted through the placenta. Macrosomia or large fetal size is directly related to maternal glucose levels. Although the phenomenon is long known, the interpretation of it being a mechanism of increasing investment in the fetus is new. Will it be possible for us to differentiate between the two views, namely, increased fetal weight as a coincidental consequence of maternal insulin resistance versus an evolved mechanism of increasing fetal investment? Although a clean choice between the two is difficult at this stage, there is some suggestive evidence. The fetal weight gain is not simply a consequence of the obesogenic physiological setup of the mother as evidenced by the study by Catalano et al. demonstrating that in normal mothers, the fetal weight gain was positively correlated with maternal weight gain, but in diabetic mothers, the increased weight of the fetus was independent of maternal weight gain [58]. Evolutionary biologists give substantial importance to parental investment. Parental investment appears to be central to the evolution of courtship displays, mating systems, and sexual dimorphism as well as biases in sex ratios at birth. Therefore, if the increased nutrient flow through the placenta in insulin-resistant mothers is an investment strategy, there should be other detectable correlated changes.
In species where monogamy is not obligate, a male’s reproductive success depends upon access to females which in turn depend upon male’s relative social status. A high-ranking male may have a large reproductive success, and a weaker male may have little if any. A female’s success depends on her absolute reproductive capacity and is little affected by the status of other females. As a result, a male’s reproductive fitness is a sigmoid function of its physical fitness, whereas a female’s curve is saturating (Fig. 9.3). It can be seen from this curve that at low level of physical fitness, females have better reproductive success than males, but at high levels of physical strength and fitness, males do better than females. Since early life development has lifetime consequences for the physique of an individual, a mother can potentially increase her net reproductive success by making careful investment choices at birth. Since a smaller or weaker male has very low chances of reproductive success, it makes no sense to make a weak male offspring. A weak female is not an outright loser like a weak male. She can reproduce as per her own absolute capacity. Therefore, a smart mother could maximize her net reproductive success by investing more in a male fetus. Alternatively, when the female is not in a capacity to invest sufficiently in the fetus, she should produce a female fetus preferentially. This is called the Trivers–Willard hypothesis of parental investment [59, 60]. If the Trivers–Willard hypothesis is true and if fetal macrosomia in diabetic mothers represents an evolved investment strategy, it should follow the predictions of the TW hypothesis. We can make two testable predictions based on this logic. The first is that the maternal insulin resistance or maternal blood sugar-induced fetal weight gain should be more pronounced for a male fetus than a female fetus. The other is that a female having higher nutritional reserves or higher plasma glucose at the time of conception should have a male-biased probability of fetal gender. The former has not been rigorously tested but is not difficult to test. There is already good amount of evidence for the latter [60–63]. Changes in glucose levels at conception have been associated with change in the sex ratios in mice. Excess glucose seems to choose male embryos [60]. One study shows that fetal sex is associated with diet at conception in humans. High preconceptional energy leads to higher number of boys suggesting a sexual dimorphism in the embryos based on the nutritional reserves [62–64]. Since humans have predominantly biparental care, the TW effect is expected to be very weak in humans. Therefore, it is unlikely that blood sugar makes any meaningful difference for a couple desirous of a baby girl or a boy, but it could be sufficient to show statistical significance to test the hypothesis.
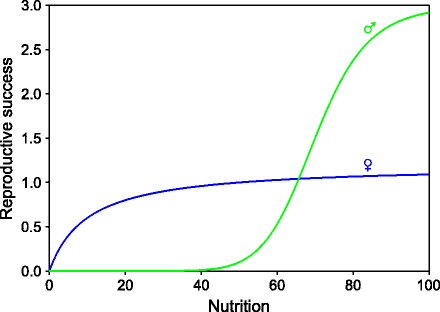

Fig. 9.3
The hypothetical differential fitness curves of males and females in promiscuous species. A female’s reproductive success is decided by her own absolute fitness, whereas a male’s reproductive success depends more on access to females which is decided by the social rank, that is, its fitness relative to other males. As a result, the difference between reproductive fitness of weaker and stronger males is very large as compared to females
The r and K selection and TW hypothesis work at the ultimate level. If the ultimate reasoning is correct, one should also find proximate mechanisms to bring about the expected adaptive changes. The next most relevant question then is at the level of endobolism: How are the r and K strategies executed? Which mechanisms lead to r reproduction and which ones lead to K? What triggers these mechanisms?
1.
Adiponectin: Adiponectin appears to be the most important player in determining reproductive strategies. This is because adiponectin affects both fecundity and transplacental nutrient transfer. It is also associated with some other parameters of reproductive strategies such as litter size and weaning to estrus interval [65, 66]. Adiponectin is important in several steps of oogenesis as well as early embryonic development [67–70]. Not surprisingly, the common ovulation disorder of modern times, PCOS, is associated with low levels of adiponectin [71–74], and it may be suspected that low adiponectin may be causal to PCOS, although this is difficult to ascertain at this stage. Adiponectin receptors are abundant in testes indicating that in males, it is likely to be playing a crucial role in spermatogenesis [75–77]. Thus, adiponectin appears to enhance the probability of conception by multiple ways. As expected by the r and K selection theory, an agent that increases the number of offspring should bring down the investment in offspring simultaneously. Adiponectin does this by regulating the transplacental flow of nutrients. Evidence for this is not only correlational [78, 79] but also experimental, where rats infused with adiponectin showed reduction in fetal weights [80] and lower expression of glucose transporter in the placenta [81]. This is in addition to the insulin sensitizing effects of adiponectin. By increasing insulin sensitivity of maternal tissues, it shifts the budget allocation more towards maternal tissues and less towards offspring. Limiting the investment in a given fetus can be potentially helpful in providing for subsequent pregnancies. Under conditions such as transient maternal undernutrition where nutrients need to be preferentially transported to the fetus, adiponectin is downregulated [81]. The adiponectin response to caloric restriction is likely to have primarily evolved for this purpose.
2.
Insulin and differential allocation of glucose: The placenta is an insulin-independent tissue. Therefore when maternal tissues become insulin resistant, there is increased flow of glucose through the placenta. This differential allocation of energy explains why insulin-resistant mothers have larger babies. High levels of leptin that are commonly associated with obesity also increase nutrient flow across the placenta by modulating system A transporters [82]. Not surprisingly, both obesity and insulin resistance are known to reduce fecundity. This, as opposed to adiponectin, marks an inclination towards K type of reproduction.
3.
Active role of placenta: The placenta plays an active role in drawing more nutrients to it. A hormone called human chorionic somatomammotropin is secreted by the placenta in large quantities, and this hormone induces insulin resistance in maternal tissues [83]. As a result, less glucose is taken up by maternal tissues and thereby more is diverted through the placenta. To what extent this mechanism is important in gestational insulin resistance is not known. The placenta also actively secretes adiponectin [81] which has a diametrically opposite effect. The placenta appears to do a balancing or fine-tuning act. The dynamics of this in relation to sexual behavior and reproductive strategies would potentially be of great interest but currently remains an almost virgin area for research.
4.
Investment in milk: Similar to transplacental investment, investment in lactation is also influenced by components of the insulin resistance syndrome. Adiponectin is negatively associated with the frequency and duration of lactation [84, 85]. IGF-1 which has insulin sensitizing effects is negatively associated with milk yields in cows and positively with conception indicating that it is one of the mediators of a trade-off between number of offspring and investment in each [86]. Insulin and cortisol stimulate casein synthesis in the mammary gland and thereby increase the investment in milk [87–91]. Plasma glucose levels also enhance milk production [92]. More interestingly, milk glucocorticoids also affect hawk–dove behavior in future life of infants in a gender-specific manner [93]. All these findings are in support of the hypothesis of differential investment and r and K trade-off mediated by important players of the metabolic syndrome.
5.
K strategy accompanied by longer life. Is there a contradiction? The r and K selection strategies in reproduction are also accompanied by differences in life span. The r strategists are typically short lived and K strategists long lived. In mammals, body size and longevity correlate positively with each other and negatively with the rate of reproduction [94, 95]. It makes sense therefore that insulin signaling is also connected to longevity. In the worm Caenorhabditis elegans, mutations of genes involved in insulin/IGF-1 signal transduction lead to extended life span [96, 97]. Mutations in genes homologous to the mammalian insulin/IGF-1 receptor signaling pathway, insulin receptor substrate homologue chico, or insulin-like receptor InR gene extend longevity in Drosophila [96]. In mammals and particularly humans, insulin is certainly connected to longevity but in a rather contradictory way. On the one hand, insulin resistance is associated with a series of fatal disorders, and centenarians are known to have high insulin sensitivity [96], but on the other, the klotho gene whose overexpression is known to increase life span has been shown to act by increasing insulin resistance [98]. FIRKO mice with fat cell-specific insulin resistance have a prolonged life span [99]. On the contrary, life extension in dwarf mice is associated with reduced secretion of insulin and increased hepatic sensitivity to insulin [100]. The key differences in the contradictory results might lie in differential tissue-specific resistance to insulin. Currently the association of insulin resistance with longevity in humans remains ambiguous. However, there is strong evidence that insulin signaling and insulin resistance are certainly involved in determining longevity in widely differing animals. We will come back to this paradox again in a later chapter with more clear assertion that actually insulin resistance has a pro-longevity effect, but certain problems arise in humans which make the change pathological.
There is one note of caution while using the r and K reproduction concepts in humans. Previously they have been applied to humans in a different context, with an argument that some human races are more r selected than others and that K selected races are more intelligent. This argument has a racist connotation. The argument above is driven by ecology and behavioral strategies, not by race or ethnicity. In this context, evidence that higher level of cognitive ability correlates with K strategy [101] has no racial implications. But why should K reproduction be accompanied by higher-level cognitive capacity? There is a logical reason which leads us back to the soldier–diplomat dichotomy.
Back to Soldier–Diplomat
Till the last chapter, we were talking about two alternative behavioral strategies, namely, soldier and diplomat, and arguing that diplomat behavior or deficiency of soldier behavior has a causative role in metabolic syndrome disorders. Here we are talking about two alternative reproductive strategies, namely, r and K, and contemplating that K strategy has a causal role in metabolic syndrome disorders and chronic crowding would trigger mechanisms of K reproduction. Are these two independent switches for the insulin-resistant state?
By all means, the two are not independent. Crowding or high population density affects reproductive strategies on the one hand which is more obvious. What is somewhat less obvious but well supported by both theory and empirical data is that crowding affects aggression to a considerable extent. A persistent and popular view holds that crowding leads to violence. Experimental data from free-ranging and captive animals is contradictory and so are data on humans. Some studies demonstrate increase in aggression upon crowding [102–109], and others report a net decrease with crowding [110–116]. Social isolation on the other hand is more consistently known to induce aggression and is a typical model of inducing aggression in rodent experiments [117–120]. This inconsistency comes at least partially from the different experimental designs and the history of animal groups under study. Crowding has a multitude of social effects too [121], and they may prevail over the actual effects of crowding on aggression. For example, if crowding is experimentally increased by increasing the number of animals, it inevitably means some unfamiliar individuals have to interact, and this creates a different social situation. The effects of a sudden change also need to be differentiated from the effects of crowding. For example, in one study, intense fighting and killing ensued when a group of macaques was released in a larger space. When after two and half years the same group was crowded back into a smaller pen, a similar bout of aggression was observed again [116]. This appears to be simply a response to change than a response to crowding.
Another important reason for the contradictory results is the lack of an appropriate null model for a fair comparison. The question relevant to us here is not whether the total incidents of aggression increase but whether individuals tend to behave more aggressively upon exposure to crowding. So the total frequency of crowding is not directly relevant. Many studies report mean per capita aggressive encounters. This also does not reflect on an individual becoming more aggressive. It should be noted that as crowding increases, the potential number of conflict situations would increase for an individual. If we assume that individuals interact with each other randomly, then the total number of encounters should increase in square proportion of crowding. The per capita encounters should increase in direct proportion to population size. In reality, this does not happen because there is some social and spatial viscosity. Any individual does not have equal probability of interacting with all other individuals. Therefore, in reality, the total number of potential encounters would increase in a saturation curve rather than in square proportion. This curve should be the null model in assessing the effects of crowding on aggression. Unfortunately, it is not easy to work out this saturation curve making it difficult to assess the effects of crowding on the frequency of aggression. In experiments where crowding appears to decrease the frequency of total aggressive encounters instead of increasing it, the interpretation is clear and robust. In studies where there is a marginal increase in the total number of aggressive encounters, after applying the null model curve even conceptually, the inference may reverse in most of the studies. This makes the evidence for pro-aggression effects of crowding substantially weaker [103, 122].
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree


