(1)
Mathematics in Naples, Naples, Italy
Deceased
In any movement inside a fluid, a resistance is exerted. Therefore, the natural degree of impetus which should carry the mobile is weakened. Consequently, the unimpeded degrees of velocity, i.e. in vacuum, which are naturally displayed by heavy bodies are necessarily quicker and more impetuous than those which are exerted in fluid environments. But nevertheless these bodies have no infinite velocity and impetus. They have a certain and well-determined degree of velocity assigned to them by nature. This velocity is not instantaneous. The famous demonstration of Aristotle actually opposes this opinion. He asserted that movement should not occur in vacuum over some time but instantaneously. It is thus worthwhile examining the reasoning of Aristotle which results from this hypothesis.
He claims that, whenever the same mobile is moved through two fluid environments, their densities or resistances are inversely proportional to the velocities of the mobile in these fluids. Let us suppose that an iron ball for example is moved through water and through air by one and the same motive force from its natural disposition. If the density and resistance to division of water was 100 times greater than the resistance of air, the philosopher assumes that the iron ball moves through air at a velocity 100 times greater than through water. If the movements occur in equal times, the distance travelled through air will be 100 times that travelled through water. If the travelled distances are equal, the duration of the motion through water will be 100 times slower and longer than through air.
Based on this principle, the philosopher claims that the velocity of any mobile in vacuum is tremendous and instantaneous. Of course this reasoning would be excellent if the said principle assumed by the philosopher was firm and well established. But the famous Galileo already demonstrated that it is obviously false, in his first dialogue on the new science of mechanics.
Proposition CCIII
With our new demonstration we will show that in two fluid environments unequally dense and resisting, the ratio of the velocities of the same heavy body can be larger or smaller than the inverse ratio of the densities of the fluids if, however, the heavy body falls in both fluids.
In a vessel CF let be a fluid M the density, thickness or resistance to division of which has a certain and well-determined magnitude which is S (Table 10.1, Fig. 1). In the vessel CG let be another fluid N the density and resistance R of which is greater than S. Moreover, the same mobile able to fall in both fluids M and N, travels a distance CD in the fluid M or a distance CE in the other fluid N in the same time T. The motive force of the mobile A is one and has a certain and well-determined magnitude. Therefore, the natural impetus and velocity of the heavy body A would be always the same and have the same magnitude if all the obstacles which are produced by the resistance of the environment could be removed, since the velocity of the heavy body A in the different fluids M, N is altered and modified by no other cause than a difference in the resistance of the fluids which modify the natural impetus and movement of the mobile. Consequently, let us suppose that the absolute magnitude of the velocity of the heavy body A neither slowed down nor impeded by the density of some fluid environment is such that in the time T the mobile can travel a longer distance CL. Therefore, the slowing down provoked by the density of the fluid M impeding the movement of the heavy body is DL. But the heavy body is slowed down by the higher density R of the other fluid N and a distance EL longer than DL is subtracted from its whole natural course. But the non travelled distance DL resulting from the density S of the fluid M is smaller than the distance CE travelled in the fluid N at a lower velocity. I claim that the ratio of the greater velocity of the body A in the fluid M to its lesser velocity in the fluid N is less than the ratio of the resistance or density R to the resistance S. If DL is equal to CE they are proportional. But if DL is greater than CE, the ratio of the velocity of A in M to its velocity in N is greater than the ratio of the densities R/S.
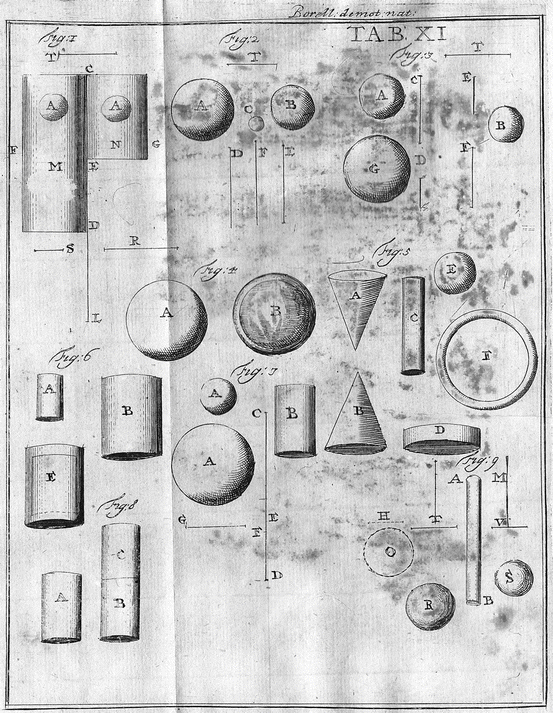
Table 10.1

Let us suppose at first that DL is smaller than CE. The ratio of ED to the larger CE is smaller than its ratio to the smaller DL. Consequently, by combining, the ratio DC/CE is smaller than EL/LD. But DC/CE is equal to the ratio of the velocity of A in the fluid M to its velocity in the fluid N (because, in the same time, the velocities are exactly proportional to the travelled distances). Similarly, the ratio of the impedance and slowing down which the density R of the fluid N imposes on the movement of the body A to the slowing down imposed by the density S of the fluid M in the same time is equal to the ratio of the distances EL/DL which are the not travelled distances in these fluids. Consequently, the ratio of the velocity of the body A in the fluid M to its velocity in the fluid N is smaller than the ratio of the density and resistance of the fluid N to the density of the other fluid M.
Then DL is supposed to be equal to CE. The ratios of ED to two equal quantities are equal. By combining, DC/CE = EL/LD. Therefore, the ratio of the densities R/S is equal to the ratio of the velocity of the body A in M to its velocity in the fluid N.
Finally, DC is supposed to be greater than CE. Consequently, DC/CE is greater than EL/LD and, therefore, the ratio of the velocity of A in M to its velocity in N is greater than R/S, i.e. than the ratio of the density of the fluid N to the density of the fluid M.
Corollary
Consequently, it is not true that the velocities of the same heavy body in two fluid environments are always inversely proportional to the resistances of the fluids.
Let us indeed suppose a golden globe falling in an empty space at its absolute and unaltered natural velocity, all obstacles being removed, so that it travels a height of 100 cubits in 1 s. In the same time, it travels a distance of 90 cubits by falling in water, and 30 cubits in quicksilver so that its velocity in water is thrice that in quicksilver. But calculation shows that the density of quicksilver is not three times but seven times the density of water.
Then two other more different fluid environments are taken. In the same time the golden globe travels 80 cubits in the rarer, 20 in the denser. The ratio of the densities of the fluids then is exactly four times the ratio of the velocities. Finally, in less different fluids, if the ratio of the velocities is two, that of the resistances is three. The hypothesis of Aristotle thus appears to be wrong. Consequently, the velocity of any heavy body in an empty space is not instantaneous. And of course, if we consider the nature of movement, which cannot be conceived without a succession of local passages, we can certainly not conceive that a finite body moves in the instant from one place to another. It would indeed be altogether at the starting and at the arriving point of its movement and thus would occupy a distance longer than itself. Moreover, the concept of successive displacements from one place to another would be eliminated. Consequently, any finite body impelled by a finite motive virtue, even if all obstacles are removed from the fluid environment, must travel a certain distance in a certain well-determined time. But this will be shown more extensively and more accurately below.
It must be found out whether all natural bodies must move in the same empty space at equal or at unequal velocities. At a first glance, it seems unbelievable and absurd that all of them must be equally rapid. In the displacements of natural bodies the ratio of the faculties of carrying out a movement must be known above all. These unquestionably result from the forces of gravity of the bodies and these forces appear to be very unequal. Therefore, the impulses and velocities which result from them will also be unequal. This was asserted by Aristotle here and there in his Physics and On Heaven. It is thus worth demonstrating the absurdity of his proposition. He thus says that heavy bodies move according to the ratio of their gravities and that light bodies also have velocities proportional to their lightness. What is even more surprising, he says he has observed this, and that it appears to the senses. Two unequal volumes of the same body, for example air, rise at unequal velocities and proportionally to their magnitudes. That is the reason, he says, why we see two unequal masses of earth (as long as they are similar otherwise) fall, the bigger one more quickly than the smaller, proportionally to their sizes. This is absolutely untrue as shown by the evidence of the senses. Two iron balls are unequal, one weighing 100 oz and the other one. For convenience, they have the same spherical shape and the density of their substance is uniform and homogeneous. These balls are dropped from a height of 100 cubits so that the ratio of their velocities is equal to the ratio of their gravities or sizes. After the bigger ball has travelled all the height of 100 cubits, the other ball of 1 oz must have travelled 1 cubit only. Therefore, this will be at an altitude of 99 cubits from the surface of the earth when the other ball has reached the earth. Aristotle says that this is what appears or is seen. But this disagrees completely with experience. Indeed, it is clear that a fairly small difference appears between the falls of these bodies. The same is observed with inhomogeneous and dissimilar bodies which have different specific weights, such as would be two balls equal in size, one of wood, the other of iron. Although these have similar and equal figures, the ratio of their velocities during their fall is not equal to the ratio of their weights, as Aristotle thought. They actually fall at about the same velocity.
But it is not beyond the scope of our matters to show the shortcoming of the reasoning of Aristotle and to indicate the main cause of his mistake. He says indeed that the downwards movement results from the force of gravity as the effective cause. Therefore, unequal gravities must also result in unequal velocities of displacement.
Proposition CCIV
Unequal weights do not produce unequal velocities but one and the same velocity.
This results from what was said in our book On the Force of Percussion. The velocities of two bodies are not measured by their weights, in that their velocities are proportional to their weights. Bodies the gravity of which are very different, can fall at one and the same velocity because the very small material particles of their substance must be supposed to be equally heavy and, added together, they cannot increase the velocity since one is not able to impel the other. All have equal motive forces, and an equal force cannot act on its equal and, therefore, will not move it forwards. Consequently, the velocity will not be increased. Similarly, ten sporting dogs equally rapid, when harnessed and running together, will not travel a longer distance in the same time than one of them. Therefore, even if the material mass is increased, and however much the weight increases and multiplies, the motive force does not increase in intensity but only in expansion inasmuch as it expands in an uniform distribution in all the particles of the heavy substance. They thus cannot increase the velocity. Moreover, it is untrue that the velocities of the falls are proportional to the weights of unequal bodies. From this hypothesis it is evidently concluded that a heavier body falls more slowly than a lighter one.
This was very elegantly demonstrated by Galileo in his first dialogue on the new science of mechanics.
But, although what was said hitherto clearly shows that the ratio of the velocities of falling bodies is not equal to the ratio of their gravities, there is, however, a famous gentleman who attempts at supporting the peripatetic opinion. Indeed, he says:
I.
It is established that the virtue of gravity is the effective cause of the fall of heavy bodies. Since a downwards movement cannot possibly occur without any velocity, the same gravity which produces the fall is also the effective cause of the velocity which is part of its natural descent. Since the degree of gravity is not unique but can increase and decrease ad infinitum, it is impossible that the eminently diverse and unequal degrees of velocity produce the same effect, namely exactly the same velocity. Nor does it seem possible to deny the first and best known principles according to which, among all the virtues and faculties which can produce some effects, that which has more force produces the greater effect. Then he continues:
II.
The experiment with a weight set in one scale of a balance shows that the one which is on the opposite side rises more quickly than if the inequality was smaller or rotates more quickly where the heavy weight to revolve the machine is hung. The functioning of a clock also becomes quicker by the addition of such a weight.
He says that experience does not teach us that the shortness of the oscillation in a clock does not result from a lack of gravity. But he cannot provide a substantial explanation of this assertion.
Finally he claims that the argument of Aristotle is very strong. Indeed, since gravity overcomes the resistance of the environment in a certain proportion, it follows that the proportion between gravity and environment can be multiplied endlessly. Therefore, if a body is supposed to fall an imaginary distance at a certain degree of velocity, pushed by its gravity, there can in no way be given a body the proportion of which in relation to the actual environment is such that it traverses this environment at an even velocity. But the distance between the resistance of the imaginary distance compared with another body which is supposed to move in this environment at an equal velocity, is infinite. Anybody will immediately say that this is very absurd.
Thus, after returning the formulation of the argument: since the resistance of the environment does slow down the gravity and the heavy body moves more quickly where it meets less resistance, there is no proportion between the (add full) environment and vacuum, it necessarily follows that there is no proportion either between the time in which a heavy body travels a well-determined quantity of the environment and the time in which it travels as much in vacuum. Therefore, this distance in vacuum is travelled instantaneously.
I answer briefly to the first objection. It is not true that the effect of a greater velocity results from the virtue of a greater gravity, as if it were the effective cause, in the process itself of falling. As we showed in Prop. 20, 21 and 204, equal parts of the same heavy body by their nature must flow downwards at the same velocity. Therefore, an upper part attempts at compressing a lower one at the same velocity as that at which the lower escapes the blow. Therefore, the gravity of the upper one does not increase the compressive force or the gravity of the lower. Thus the weight of one part acts as if it were equal to the weight of the aggregate of all the parts. Thus, in a downwards movement, any unequal bodies can be considered as equally heavy. Therefore, they will not fall at equal velocities. It is not new that the force and energy of ten men can sustain a heavier weight, ten times as much, than one of them. It does not result from this that these men can run while carrying the same weights at a velocity ten times greater than one of them. Thus, although the velocity of the run results from the force and energy of these men, this velocity is not increased nor multiplied in accordance with the multiplication of men. The weakness of this first objection thus appears.
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree


